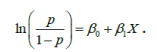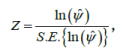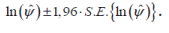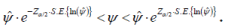Keywords
Statistics in Medicine; Epilepsy; Risk factors for Epilepsy; Odds ratio
Introduction
Epilepsy is a common chronic neurological disorder characterized by recurrent unprovoked seizures [1]. Epilepsy is a common disabling condition, which affects approximately 3% of the world population during their lifetime. About 50 million people worldwide have epilepsy, with almost 90% of these people being in developing countries [2]. Epilepsy is more likely to occur in young children or people over the age of 65 years; however it can occur at any time. Epilepsy is usually controlled, but cannot be cured with medication, although surgery may be considered in difficult cases. Socioeconomic factors may influence the risk of the disease in many ways [3]. Some studies have found increased risks of cerebrovascular disease, brain tumors, and neurodegenerative disease among people who have occupational exposure to chemicals [4], but few studies have reported on the association between specific occupations and incidence of epilepsy [5]. Socioeconomic status and occupation sometimes carry a significantly increased risk of hospitalization for epilepsy [6]. Low income and low education are associated with an increased risk among both men and women [7]. Risk is increased for men and women in certain occupational groups [8]. Over 30% of people with epilepsy do not seizure control even with the best available medications [9-11]. Epilepsy surgery is an option for patients whose seizures remain resistant to treatment with anticonvulsant medications who also have symptomatic localization-related epilepsy; a focal abnormality that can be located and therefore removed. The goal for these procedures is total control of epileptic seizures [12]. In most cultures, persons with epilepsy have been stigmatized, shunned or even imprisoned. Stigma continues to nowadays, in both the public and private spheres, but polls suggest it is generally decreasing with time, at least in the developed world [13].
Many jurisdictions forbid certain activities to persons suffering from epilepsy. The most commonly prohibited activities involve operation of vehicles or machinery or other activities in which continuous vigilance is required. However, there are usually exceptions for those who can prove that they have stabilized their condition. There is an outgoing debate in bioethics over who should bear the burden of ensuring that an epilepsy patient does not drive a car or fly an airplane [2].
Several studies have found that fatalities caused by seizures that occurred while driving were relatively rare [13-15].
Materials and Methods
Research Methodology
A total of 700 patients (512 males and 188 females) were examined. The risk factors of epilepsy investigated. We considered the sex (sex = 0, if patient is female and sex = 1, if patient is male). This study has a number of strengths. For example, our study population included a well-defined open cohort of the entire population of Greece. The present study also has some limitations. Although the national database includes data on the entire population, it only incorporates information about hospital admissions. Data on out- patient treatment are rather unavailable. Another limitation is that we were unable to test the validity of the epilepsy diagnoses. However, it seems likely that the diagnoses are valid. Moreover, to help counter any potential validity problems, we only used main diagnoses of epilepsy recorded in the hospital registers, i.e., instances in which the main cause of hospitalization was epilepsy. This increased the possibility that the diagnoses of epilepsy were valid.
It is sometimes helpful to describe the chance that a binary response variable leads to a success in terms of the odds of that event. When two sets of binary data are to be compared, a relative measure of the odds of success in one set relative to that in the other is referred to as the odds ratio
Suppose a (2×2) table is given as
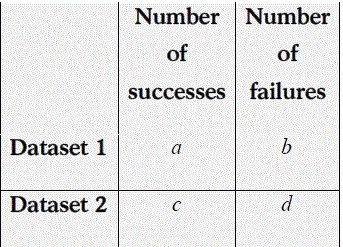
Then the odds ratio is given as:
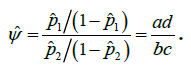
This estimate is the ratio of the products of the two pairs of diagonal elements in the above 2×2 table, and for this reason, 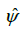 is sometimes referred to as the cross-product ratio. The association between two factors can be tested via odds ratio. The null hypothesis to be tested in this case is:
is sometimes referred to as the cross-product ratio. The association between two factors can be tested via odds ratio. The null hypothesis to be tested in this case is:
H0 :ψ = 1
or equivalently:
H0 :ln(ψ) = 1
Note that testing above hypothesis is same as testing:a
H0 :β1 = 0
in simple logistic model:
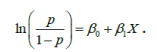
The null hypothesis:
H0 : ψ = 1
or:
H0 :ln(ψ) = 0
may be tested using the test statistic:
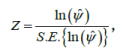
which has an approximate standard normal distribution. An approximate 100(1-α)% confidence interval for ln(ψ) is constructed as:

For example, a 95% confidence interval
for ln(ψ) is given by:
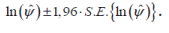
The confidence interval given by equation (2.1) on inversion will give us the confidence interval for ψ as:
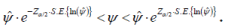
If the interval contains unity, it indicates independence; otherwise an association is indicated.
Results
Odds Ratio Analyses
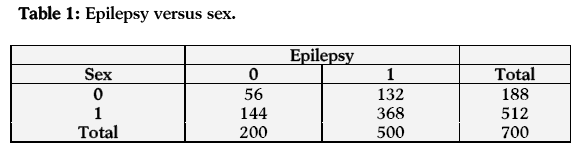
In the first case (relationship between sex and epilepsy) the estimated odds of having epilepsy are 1.084 times more for males as compared to females. Hence the males are more likely to have epilepsy than females. The estimated log odds ratio is 0.081 and its asymptotic standard error is 0.187, which is highly significant at 1% level of significance. A 95% confidence interval for the true log odds ratio is (-0.286, 0.448) and so the 95% confidence interval for the true odds ratio is (0.751, 1.564). The confidence interval shows a significant relationship between sex and epilepsy (Table 1).
In the next case (relationship between injury and epilepsy) the estimated odds of having epilepsy are 11.044 times more for persons with injury than those who do not have injury. The estimated log odds ratio is 2.402 and its asymptotic standard error is 0.397, which is highly significant at 1% level of significance. A 95% confidence interval for the true log odds ratio is (1.624, 3.180) and hence the 95% confidence interval for the true odds ratio is (5.072, 24.047). The confidence interval shows a significant relationship between injury and epilepsy (Table 2).
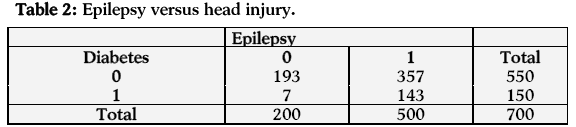
In the case of the relationship between epilepsy and meningitis, the estimated odds of having epilepsy are 4.057 times more for those who have meningitis as compared to those who have not. The estimated log odds ratio is 1.400 and its asymptotic standard error is 0.306, which is highly significant at 1% level of significance. A 95% confidence interval for the true log odds ratio is (0.800, 2.000) and hence the 95% confidence interval for the true odds ratio is (2.227, 7.391). The confidence interval shows a significant relationship between epilepsy and meningitis (Table 3).
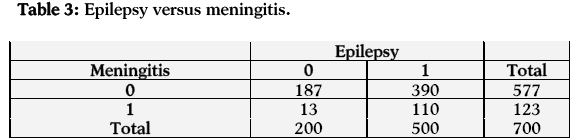
In the case of family history and epilepsy the estimated odds of having myocardial infarction are 3.266 times more those who have a family history of epilepsy as compared to those who do not have family history of epilepsy. The estimated log odds ratio is 1.184 and its asymptotic standard error is 0.231, which is highly significant at 1% level of significance. A 95% confidence interval for the true log odds ratio is (0.731, 1.637) and hence the 95% confidence interval for the true odds ratio is (2.077, 5.136). The confidence interval shows a significant relationship between family history and epilepsy (Table 4).

In the last case (relationship between age and epilepsy) the estimated odds of having epilepsy are 0.747 times more for persons having age less than 60 years than those having age equal to or greater than 60 years. Hence the persons having age equal to or greater than 60 years are more likely to have epilepsy than those who have age less than 60 years. The estimated log odds ratio is -0.292 and its asymptotic standard error is 0.188, which is highly significant at 1% level of significance. A 95% confidence interval for the true log odds ratio is (-0.660, 0.076) and so the 95% confidence interval for the true odds ratio is (0.517, 1.080). The confidence interval shows a significant relationship between age and epilepsy (Table 5).

Discussion and Conclusions
In our research study, we had no data on individual risk factors for epilepsy, however, we did adjust for socioeconomic status, which is associated with several individual risk factors for cardiovascular disease and Alzheimer’s disease, many of which (like low education and socioeconomic deprivation) are also risk factors for epilepsy.16-18A potential limitation is that there have been large changes in the labor market in Greece during the study period. Lack of information on duration of employment was partly remedied by the analysis of individuals who maintained the same occupation through two consecutive censuses. The results showed that the proportion of concordant occupational titles was 72%, suggesting a reasonable quality for the census data. Moreover, it is important to compare consistency within this study and between studies, as well as biological plausibility, before inferring causality. In addition, early onset may influence a person’s choice of occupation, which may in turn have influenced the results. Low socioeconomic status is a risk factor for the development of epilepsy. Low socioeconomic status is associated with social and economic deprivation, unemployment, and low income, which in turn are associated with risk factors like incidence of birth defects, trauma, infection, and poor nutrition [19]. Low socioeconomic status may influence the risk of epilepsy through risk factors that are the same for epilepsy as for injury, cardiovascular disease, and Alzheimer’s disease. For unskilled workers, the increased risk of epilepsy might be traceable, at least in part, to short duration of employment and associated lifestyle factors. Alcohol intake or abuse is a risk factor for epilepsy, so higher alcohol consumption in certain occupational groups may help explain raised risk of epilepsy in those groups. There is a striking similarity in the list of occupational groups with high prevalence of alcohol consumption and occupational groups with raised risk of epilepsy [20]. It is also possible that drivers’ higher risk of accident injuries may partly lie in the causal pathway: seizures are a common complication of head injuries. It is noteworthy that increased risks were found for male launderers and dry cleaners. Chemical exposures occur frequently in these occupations; for example, exposure to solvents and chemical cleaning agents. Earlier epidemiological studies have reported that solvent exposure increases the risk of epilepsy. We have no information about exposure to specific chemicals, so it is hard to identify which kind of chemical agent may have been causally associated with raised risk of epilepsy. Increased risks for these workers are not consistent in different sexes and cohorts [21-23]. These inconsistencies may partly be explained by men and women working at different types of tasks and different sites, which may dilute the risk estimation. Pathways may include exposure to organic solvents and other chemicals (such as that experienced by launderers and dry cleaners) and high alcohol consumption. However, our findings do not allow inferences about causal relationships, so the findings remain tentative [24-26].
5309
References
- Adelöw C, Ahlbom A, Feychting M, Johnsson F, Schwartzbaum J and Tomson T. Epilepsy as a risk factor for cancer. J NeurolNeurosurg Psychiatry 2006;77(6):784-786.
- 2.Ben-Manachem E. Toward a more pragmatic view of driving and epilepsy. Epilepsy curr 2004;4(4):133-134.
- World Health Organization (WHO). Epilepsy: Etiology, epidemiology and prognosis, 2001.
- Frigerio R, Elbaz A, Sanft KR, Peterson BJ, Bower JH, Ahlskog JE, et al. Education and occupations preceding Parkinson disease: a population-based case-control study. Neurology. 2005;65:1575–83.
- 5.Denays R, Kumba C, Lison D, De Bels D. First epileptic seizure induced by occupational nickel poisoning. Epilepsia 2005;46:961–2.
- Dockerty JD, Draper G, Vincent T, Rowan SD and Bunch KJ. Case-control study of parental age, parity and socioeconomic level in relation to childhood cancers.Int J Epidemiol 2001;30(6):1428-1437.
- Gaitatzis A, Carroll K, Majeed A and Sander J. The epidemiology of the comorbidity of epilepsy in the general population.Epilepsia 2004;45:1613-1622.
- 8.Hauser WA, Annegers JF, Kurland LT. Prevalence of epilepsy in Rochester, Minnesota: 1940-1980. Epilepsia 1991;32:429-445.
- Fisher R, Van Emde Boas W, Blume W and ElgerC.. Epileptic Seizures and Epilepsy: Definitions proposed by the (ILAE) and the international Bureau for Epilepsy (IBE). Epilepsia 2005;46(4):470-472.
- Gruman J, von Korff M, Reynolds J, Wagner EH. Organizing Health care for people with seizures and epilepsy. J Ambul Care Manage 1998;21:1-17.
- Henry TR, Drury I. Non-epileptic seizures in temporal lobectomy candidates with medically refractory seizures. Neurology 1997;48:1374-1382.
- Hesdorffer DC, Ludvigsson P, Olafsson E, Gudmundsson G, Kjartansson O, Hauser WA. ADHD as a risk factor for incident unprovoked seizures and epilepsy in children. Arch Gen Psychiatry 2004;61(7):731-736.
- Jilek-Aall L. MorbusSacer in Africa: Some religion aspects of epilepsy in traditional cultures. Epilepsia 1999;40(3):382-386.
- Johnston A, Smith P. Sudden unexpected death in epilepsy. Expert Rev Neurother 2007;7:1751-1761.
- Kanner AM, Balabanov A. Depression and epilepsy: How closely related are they? Neurology 2002;58:S27-S39.
- Ludvigsson P, Hesdorffer D, Olafsson E, Kjartansson O, Hauser WA. Migraine with aura is a risk factor for unprovoked seizures in children. Ann Neurol 2006;59(1):210-213.
- Murphy JM, Horton NJ, Monson RR, Laird NN, Sobol AM, Leighton AH. Cigarette smoking in relation to depression: Historical trends from the Stirling County Study. Am J Psychiatry 2003;160:1663-1669.
- Park JO, Shin SD, Kim J, Song KJ, Peck MD. Association between socioeconomic status and burn injury severity. Burns 2009;35(4):482-490.
- Matuja WB, Kilonzo G, Mbena P, Mwango'mbola RL, Wong P, Goodfellow P, Jilek-Aall L. Risk factors for epilepsy in a rural area in Tanzania: A community-based case-control study. Neuroepidemiology 2001;20(4):242-247.
- Pramuka M, Hendrickson R, Zinski A, Van Cott AC. A psychosocial self-management program for epilepsy: A randomized pilot study in adults. Epilepsy Behav 2007;11:533-545.
- Sidenvall R, Heijbel J, Blomquist HK, Nyström L, Forsgren L. An incident case-control study of first unprovoked afebrile seizures in children: A population-based study of pre- and perinatal risk factors. Epilepsia 2001;42(10):1261-1265.
- Thompson PJ, Upton D. The impact of epilepsy on the family. Seizure, 1992;1:43-48.
- Tromp SC, Weber JW, Aldenkamp AP, Arends J, vander Linden I, Diepman L. Relative influence of epileptic seizures and epilepsy syndrome on cognitive function. J Child Neurol 2003;18:407-412.
- Warnakulasuriya S. Significant oral cancer risk associated with low socioeconomic status. Evid Based Dent 2009;10(1):4-5.
- Wilson SJ, Bladin PF, Saling MM. Paradoxical results in the cure of chronic illness: The "burden of normality" as exemplified following seizure surgery. Epilepsy Behav 2004;5:13-21.
- Cascino G. D. Epilepsy: Contemporary perspectives on evaluation and treatment. Mayo Clinic Proc 1994;69:1199-1211.






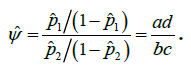
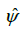 is sometimes referred to as the cross-product ratio. The association between two factors can be tested via odds ratio. The null hypothesis to be tested in this case is:
is sometimes referred to as the cross-product ratio. The association between two factors can be tested via odds ratio. The null hypothesis to be tested in this case is: